
The degrees of freedom (DF) in statistics indicate the number of independent values that can vary in an analysis without breaking any constraints. It is an essential idea that appears in many contexts throughout statistics including hypothesis tests, probability distributions, and linear regression.
| Source of Variation | Sums of Squares (SS) | Degrees of Freedom (df) |
|---|---|---|
| Between Treatmenst | 75.8 | 4-1=3 |
| Error (or Residual) | 47.4 | 20-4=16 |
| Total | 123.2 | 20-1=19 |
How many degrees of freedom in error are there?
There are 28 observations and the two independent variablesuse a total of two degrees of freedom. The output displays the remaining 26 degrees of freedom in Error. The error degrees of freedom are the independent pieces of information that are available for estimating your coefficients.
How are degrees of freedom related to sample size?
Because the degrees of freedom are so closely related to sample size, you can see the effect of sample size. As the DF decreases, the t-distribution has thicker tails. This property allows for the greater uncertainty associated with small sample sizes. The degrees of freedom chart below displays t-distributions.
Why do regression models have so many error degrees of freedom?
For precise coefficient estimates and powerful hypothesis tests in regression, you must have many error degrees of freedom, which equates to having many observations for each model term. As you add terms to the model, the error degrees of freedom decreases. You have fewer pieces of information available to estimate the coefficients.
What are the degrees-of-freedom of errors?
Every type of error has its own degrees-of-freedom associated with it. Sometimes, the mean squared errors are also called variances as discussed in later articles.
What are the degrees of freedom for the treatment and error sum of squares?
The third column contains degrees of freedom. The between treatment degrees of freedom is df1 = k-1. The error degrees of freedom is df2 = N - k. The total degrees of freedom is N-1 (and it is also true that (k-1) + (N-k) = N-1)....The ANOVA Procedure.Control(X - 1.2)(X - 1.2)20-1.21.431.83.2Totals010.63 more rows•Jan 23, 2019
What is the degree of freedom for error?
The degrees of freedom add up, so we can get the error degrees of freedom by subtracting the degrees of freedom associated with the factor from the total degrees of freedom. That is, the error degrees of freedom is 14−2 = 12.
How many degrees of freedom are there for the between groups variation?
Between Group Variation There are k samples involved with one data value for each sample (the sample mean), so there are k-1 degrees of freedom.
What is degree of freedom in analysis of variance?
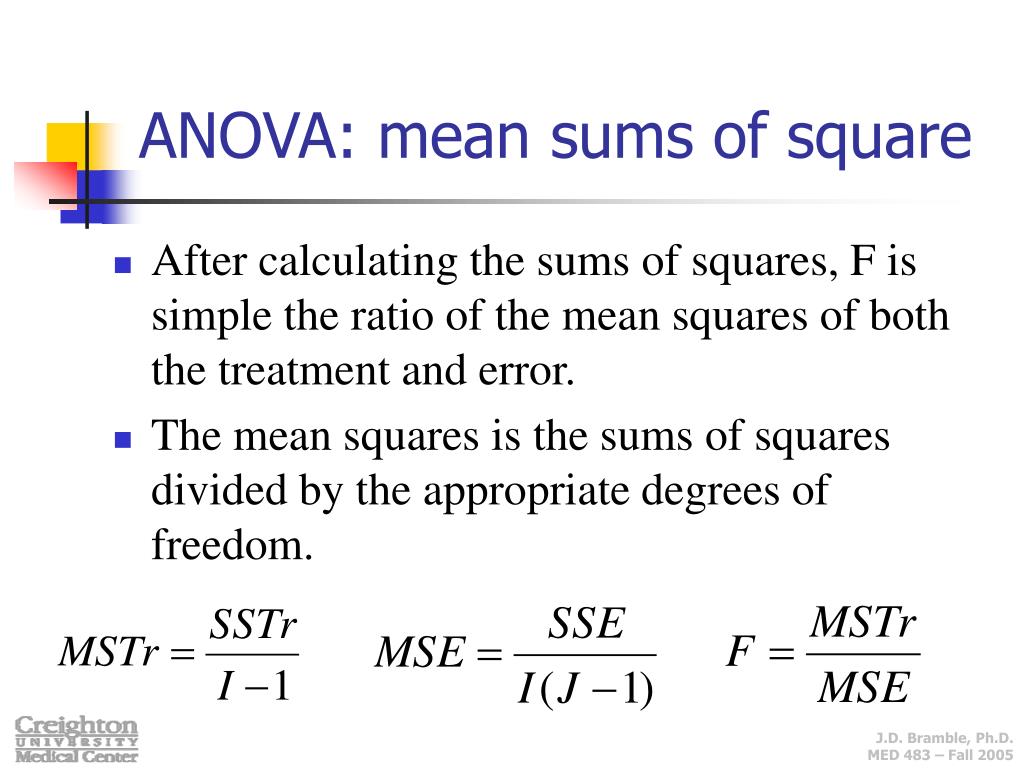
The degrees of freedom (DF) are the number of independent pieces of information. In ANOVA analysis once the Sum of Squares (e.g., SStr, SSE) are calculated, they are divided by corresponding DF to get Mean Squares (e.g. MStr, MSE), which are the variance of the corresponding quantity.
What do you mean by degrees of freedom?
Degrees of freedom refers to the maximum number of logically independent values, which are values that have the freedom to vary, in the data sample. Degrees of freedom are commonly discussed in relation to various forms of hypothesis testing in statistics, such as a chi-square.
How do you determine degrees of freedom?
To calculate degrees of freedom, subtract the number of relations from the number of observations. For determining the degrees of freedom for a sample mean or average, you need to subtract one (1) from the number of observations, n.
How do you find the degrees of freedom between groups?
The degrees of freedom within groups is equal to N - k, or the total number of observations (9) minus the number of groups (3).
How do you find the degrees of freedom for two samples?
To calculate degrees of freedom for two-sample t-test, use the following formula: df = N₁ + N₂ - 2 , that is: Determine the sizes of your two samples.
What is degree of freedom in T distribution?
The particular form of the t distribution is determined by its degrees of freedom. The degrees of freedom refers to the number of independent observations in a set of data. When estimating a mean score or a proportion from a single sample, the number of independent observations is equal to the sample size minus one.
How many degrees of freedom are there in statistics?
You end up with n - 1 degrees of freedom, where n is the sample size. Another way to say this is that the number of degrees of freedom equals the number of "observations" minus the number of required relations among the observations (e.g., the number of parameter estimates).
What is the importance of degrees of freedom?
Degrees of freedom are important for finding critical cutoff values for inferential statistical tests. Depending on the type of the analysis you run, degrees of freedom typically (but not always) relate the size of the sample.
What is degrees of freedom in regression?
Total Degrees of Freedom for Linear Regression Generally, the degrees of freedom is equal to the number of rows of training data used to fit the model. Consider a dataset with 100 rows of data as before, but now we have 70 input variables. This means that the model has 70 coefficients or parameters fit from the data.
TWO MODELS
Consider a very simple experiment of two observations, where a y variable (which may be peak heights in the presence of a baseline or constant interferent) is measured as an x variable is varied (eg, concentrations).
RESIDUAL ERRORS
Using each model, we can estimate the values of y from x or . These estimates are presented in Table 1 .
DEGREES-OF-FREEDOM
For both models, we have performed the same experiment and used the same observations.
ADDITIONAL TERMS
Models do not need to be restricted to linear terms, for example, a series of 3 observations can be used to obtain a linear model with no intercept with D = 2 degrees-of-freedom for lack-of-fit, a linear model with an intercept with D = 1, or a model including intercept, linear, and quadratic terms with D = 0.
REPLICATES
However, the measurement of residuals alone does not always tell us enough to be able to decide whether any model is significant. Imagine being told that the error in modelling a process is 0.1 AU (its response may be measured spectroscopically). On its own, this probably does not convey much, and it is a good idea to compare this to a yardstick.
SOURCES OF ERROR
Any statistical design can be analysed for different sources of error or variability.
DEGREES-OF-FREEDOM TREE
Sometimes, the degrees-of-freedom can be represented by a “degrees-of-freedom tree.” In our case, it is represented in Figure 2 and is a good way of summarising a design. Sometimes, degrees-of-freedom trees can be more elaborate, for example, if errors are viewed as coming from different sources.
What is degree of freedom?
Degrees of freedom encompasses the notion that the amount of independent information you have limits the number of parameters that you can estimate. Typically, the degrees of freedom equal your samplesize minus the number of parameters you need to calculate during an analysis. It is usually a positive whole number.
What does DF mean in statistics?
In statistics, the degrees of freedom (DF) indicate the number of independent values that can vary in an analysis without breaking any constraints. It is an essential idea that appears in many contexts throughout statistics including hypothesis tests, probability distributions, and regression analysis. Learn how this fundamental concept affects the ...
What is the difference between a MANOVA and a multivariate analysis of variance?
Multivariate analysis of variance (MANOVA) is simply an ANOVA with several dependent variables. That is to say, ANOVA tests for the difference in means between two or more groups, while MANOVA tests for the difference in two or more vectors of means.
What is the main effect of a study?
Main effect: A main effect is the effect where the performance of one variable considered in isolation by neglecting other variables in the study . Interaction: An interaction effect occurs where the effect of one variable is different across levels of one or more other variables.
What is a two way factor?
Measures 2 factors. Uses only one technician (unless technicians are one of the factors) Two-way (with replicates) Measures 2 factors, but has multiple repetitions of each combination. Uses only one technician (unless the technicians are one of the factors)
What are the advantages of MANOVA over ANOVA?
There are various advantages of MANOVA over ANOVA. Study any interaction between the factors. Study two or more factors simultaneously increase the model’s efficiency. MANOVA reduces the chances of alpha risk. Less residual variation in the model when more factors are in the study.

What Are Degrees of Freedom?
Degrees of Freedom Definition
- What are degrees of freedom in statistics? Degrees of freedom are the number of independent values that a statistical analysis can estimate. You can also think of it as the number of values that are free to vary as you estimate parameters. I know, it’s starting to sound a bit murky! DF encompasses the notion that the amount of independent information you have limits the numbe…
Independent Information and Constraints on Values
- The degrees of freedom definitions talk about independent information. You might think this refers to the sample size, but it’s a little more complicated than that. To understand why, we need to talk about the freedom to vary. The best way to illustrate this concept is with an example. Suppose we collect the random sample of observations shown below. Now, imagine we know th…
How to Find The Degrees of Freedom in Statistics
- As you can see, that last number has no freedom to vary. It is not an independent piece of information because it cannot be any other value. Estimating the parameter, the mean in this case, imposes a constraint on the freedom to vary. The last value and the mean are entirely dependent on each other. Consequently, after estimating the mean, we have only 9 independent …
Degrees of Freedom Formula
- The degrees of freedom formula is straightforward. Calculating the degrees of freedom is often the sample size minus the number of parameters you’re estimating: DF = N – P Where: 1. N = sample size 2. P = the number of parameters or relationships For example, the degrees of freedom formula for a 1-sample t test equals N – 1 because you’re estimating one parameter, th…
Df and Probability Distributions
- Degrees of freedom also define the probability distributions for the test statistics of various hypothesis tests. For example, hypothesis tests use the t-distribution, F-distribution, and the chi-square distribution to determine statistical significance. Each of these probability distributions is a family of distributions where the DF define the shape. Hypothesis tests use these distribution…
Degrees of Freedom For T Tests
- T tests are hypothesis tests for the mean and use the t-distribution to determine statistical significance. A 1-sample t test determines whether the difference between the sample mean and the null hypothesis value is statistically significant. Let’s go back to our example of the mean above. We know that when you have a sample and estimate the mean, you have n – 1 degrees o…
Degrees of Freedom Table
- You’ll often find degrees of freedom in statistical tables along with their critical values. Statisticians use the DF in these tables to determine whether the test statisticfor their hypothesis test falls in the critical region, indicating statistical significance. For example, in a t-table, you’ll find the degrees of freedom in the first column of the table. You must know the degrees of freed…
How to Find Degrees of Freedom For Tables in Chi-Square Tests
- The chi-square test of independence determines whether there is a statistically significant relationship between categorical variablesin a table. Just like other hypothesis tests, this test incorporates DF. To find the chi-square DF for a table with r rows and c columns, use this formula to calculate degrees of freedom: (r-1) (c-1). However, we can create tables to understand how t…
Linear Regression Degrees of Freedom
- Calculating degrees of freedom in linear regression is a bit more complicated, and I’ll keep it on the simple side. In a linear regression model, each term is an estimated parameter that uses one degree of freedom. In the regression output below, you can see how each linear regression term requires a DF. There are n = 29 observations, and the two independent variables use a total of tw…