You sum them quadratically: s = sqrt (s1^2 + s2^2 +... + s12^2) Conceptually you sum the variances, then take the square root to get the standard deviation.
Full Answer
How do you add up the sum of squared differences?
Here the symbol x̄ refers to the sample mean, and the symbol Σ tells us to add up the squared differences (x i - x̄) for all i . While this formula works for calculations, there is an equivalent, shortcut formula that does not require us to first calculate the sample mean. This shortcut formula for the sum of squares is
How do you find the sum of squares in a regression?
The regression type of sum of squares indicates how well the regression model explains the data. A higher regression sum of squares indicates that the model does not fit the data well. The formula for calculating the regression sum of squares is: Where: ŷ i – the value estimated by the regression line.
How do you find the sum of squared deviation?
The computed x is known as the deviation score for the given data set. Squaring each of this deviation score and then adding these squared deviation scores gave us the sum of squared deviation (SS), which is represented mathematically as. S S = ∑ ( x 2) = ∑ ( X − X ¯) 2.
How to compute the sum of squares of this data set?
To compute the sum of squares of this data set, follow these steps Calculate the average of the given data by summing all the values in the data set and then divide this sum of numbers by the total number of observations in the date set.

How do you find the sum of squares for treatment?
0:112:13The Sums of Squares Treatment in ANOVA (Module 2 2 6) - YouTubeYouTubeStart of suggested clipEnd of suggested clipSo another way we can write the sums of squares for treatment is to say the number of people in eachMoreSo another way we can write the sums of squares for treatment is to say the number of people in each group the n sub J multiplied by the deviation between the group mean for the group J.
How do you find standard deviation from sum of squares?
The formula for standard deviation is the square root of the sum of squared differences from the mean divided by the size of the data set.
How do you find the sum of squares with the sample mean and standard deviation?
The mean of the sum of squares (SS) is the variance of a set of scores, and the square root of the variance is its standard deviation. This simple calculator uses the computational formula SS = ΣX2 - ((ΣX)2 / N) - to calculate the sum of squares for a single set of scores.
How do you find the sum of standard deviations?
The standard deviation formula may look confusing, but it will make sense after we break it down. ... Step 1: Find the mean.Step 2: For each data point, find the square of its distance to the mean.Step 3: Sum the values from Step 2.Step 4: Divide by the number of data points.Step 5: Take the square root.
Standard Formula Example
To see how this shortcut formula works, we will consider an example that is calculated using both formulas. Suppose our sample is 2, 4, 6, 8. The sample mean is (2 + 4 + 6 + 8)/4 = 20/4 = 5. Now we calculate the difference of each data point with the mean 5.
Shortcut Formula Example
Now we will use the same set of data: 2, 4, 6, 8, with the shortcut formula to determine the sum of squares. We first square each data point and add them together: 2 2 + 4 2 + 6 2 + 8 2 = 4 + 16 + 36 + 64 = 120.
How Does This Work?
Many people will just accept the formula at face value and do not have any idea why this formula works. By using a little bit of algebra, we can see why this shortcut formula is equivalent to the standard, traditional way of calculating the sum of squared deviations.
Is It Really a Shortcut?
It may not seem like this formula is truly a shortcut. After all, in the example above it seems that there are just as many calculations. Part of this has to do with the fact that we only looked at a sample size that was small.
What does a lower residual sum of squares mean?
Generally, a lower residual sum of squares indicates that the regression model can better explain the data while a higher residual sum of squares indicates that the model poorly explains the data.
Why is sum of squares important?
In finance, understanding the sum of squares is important because linear regression models. Forecasting Methods Top Forecasting Methods. In this article, we will explain four types of revenue forecasting methods ...
What does a higher sum of squares mean?
A higher regression sum of squares indicates that the model does not fit the data well.
Why use standard deviation?
You can use the standard deviation formula to find the annual rate of return of an investment or study an investment's historical volatility. Investment firms can use standard deviation to report on their mutual funds and other products as it shows whether the return on funds is deviating from normal expectation.
How to find the mean of a data set?
You can find the mean, also known as the average, by adding up all the numbers in a data set and then dividing by how many numbers are in the whole set. The data set for this example problem is 6, 8, 12, 14. Add all the numbers in the data set, then divide by 4 for an average of 10.
What is the RSD in statistics?
Relative standard deviation, or RSD, is a special form of standard deviation that, in certain circumstances, is more convenient. You frequently use it in statistics, probability theory, chemistry and mathematics. It is useful to businesses when comparing data such as in financial settings like the stock market.
What is standard deviation in 2021?
Standard deviation is a common mathematical formula used to measure how far numbers are spread out in a data set compared to the average of those numbers. While students use this formula in statistics and probability theory, the field of finance uses the standard deviation formula regularly to assess risk, ...
SSR, SST & R-Squared
R-squared, sometimes referred to as the coefficient of determination, is a measure of how well a linear regression model fits a dataset. It represents the proportion of the variance in the response variable that can be explained by the predictor variable.
Calculate SST, SSR, SSE: Step-by-Step Example
Suppose we have the following dataset that shows the number of hours studied by six different students along with their final exam scores:
Additional Resources
You can use the following calculators to automatically calculate SST, SSR, and SSE for any simple linear regression line:
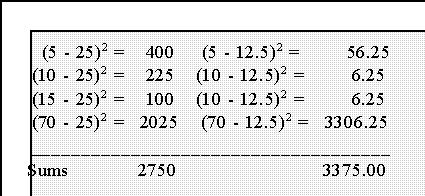
Standard Formula Example
Shortcut Formula Example
- Now we will use the same set of data: 2, 4, 6, 8, with the shortcut formula to determine the sum of squares. We first square each data point and add them together: 22 + 42 + 62 + 82= 4 + 16 + 36 + 64 = 120. The next step is to add together all of the data and square this sum: (2 + 4 + 6 + 8)2= 400. We divide this by the number of data points to obt...
How Does This Work?
- Many people will just accept the formula at face value and do not have any idea why this formula works. By using a little bit of algebra, we can see why this shortcut formula is equivalent to the standard, traditional way of calculating the sum of squared deviations. Although there may be hundreds, if not thousands of values in a real-world data set, we will assume that there are only t…
Is It Really A Shortcut?
- It may not seem like this formula is truly a shortcut. After all, in the example above it seems that there are just as many calculations. Part of this has to do with the fact that we only looked at a sample size that was small. As we increase the size of our sample, we see that the shortcut formula reduces the number of calculations by about half. We do not need to subtract the mean …