anova why sum of squares treatment

by Dr. Kaden Bechtelar I Published 3 years ago Updated 2 years ago

In ANOVA we partition the total sum of squares (TSS) in: Sums of squares due to treatments (SSTreat): measures variation due to differences between treatments (explained variation).

The SS in a can be split up into two components, called the "sum of squares of treatments" and "sum of squares of error", abbreviated as SST and SSE. where k is the number of treatments and the bar over the x.. denotes the "grand" or "overall" mean. Each n_i is the number of observations for treatment i.

Full Answer

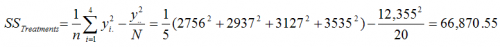
How do you find the treatment mean square in ANOVA?

Jun 22, 2021 · In the context of an ANOVA, a treatment refers to a level of the independent variable included in the model. Why do we calculate sum of squares? Besides simply telling you how much variation there is in a data set, the sum of squares is used to calculate other statistical measures, such as variance, standard error, and standard deviation.

What is the sum of squares in ANOVA?

In analysis of variance (ANOVA), the total sum of squares helps express the total variation that can be attributed to various factors. For example, you do an experiment to test the effectiveness of three laundry detergents. The total sum of squares = treatment sum of squares (SST) + sum of squares of the residual error (SSE)

What is the sum of squares for the treatment?

4 rows · This result is called the sums of squares decomposition formula. We use these results to build our ...

What is the goal of a 2-way ANOVA?

Feb 27, 2020 · What is sum of squares in Anova table? In the context of ANOVA, this quantity is called the total sum of squares (abbreviated SST) because it relates to the total variance of the observations. Thus: The denominator in the relationship of the sample variance is the number of degrees of freedom associated with the sample variance.

Why do we use sum of squares in ANOVA?

Sum of squares in ANOVA

In analysis of variance (ANOVA), the total sum of squares helps express the total variation that can be attributed to various factors. For example, you do an experiment to test the effectiveness of three laundry detergents.

What is the purpose of the sum of squares?

The sum of squares measures the deviation of data points away from the mean value. A higher sum-of-squares result indicates a large degree of variability within the data set, while a lower result indicates that the data does not vary considerably from the mean value.

What is sum of squares between in ANOVA?

The Mean Sum of Squares between the groups, denoted MSB, is calculated by dividing the Sum of Squares between the groups by the between group degrees of freedom. That is, MSB = SS(Between)/(m−1).

Which sum of squares measure the treatment effect?

The within-treatments sum of squares measures treatment effects as well as random_ unsystematic differences within each of the samples assigned to each of the treatments_ These differences represent all of the variations that could occur in study; therefore_ they are sometimes referred to as error: In ANOVA, the test ...

What is SS treatment?

Part of a video titled The Sums of Squares Treatment in ANOVA (Module 2 2 6) - YouTube

0:08

2:13

So another way we can write the sums of squares for treatment is to say the number of people in eachMoreSo another way we can write the sums of squares for treatment is to say the number of people in each group the n sub J multiplied by the deviation between the group mean for the group J.

Why do we square the residuals?

By squaring the residual values, we treat positive and negative discrepancies in the same way. Why do we sum all the squared residuals? Because we cannot find a single straight line that minimizes all residuals simultaneously. Instead, we minimize the average (squared) residual value.Nov 12, 2019

What is the difference between sum of squares and variance?

The variance of a quantity is related to the average sum of squares, which in turn represents sum of the squared deviations or differences from the mean.

What is SS and MS in ANOVA?

Each mean square value is computed by dividing a sum-of-squares value by the corresponding degrees of freedom. In other words, for each row in the ANOVA table divide the SS value by the df value to compute the MS value.Aug 30, 2021

What is sum of squares in statistics?

In statistical data analysis the total sum of squares (TSS or SST) is a quantity that appears as part of a standard way of presenting results of such analyses. It is defined as being the sum, over all observations, of the squared differences of each observation from the overall mean.

What is ANOVA treatment?

In the context of an ANOVA, a treatment refers to a level of the independent variable included in the model.

What is a treatment in an ANOVA process?

The definition is: A treatment is a specific combination of factor levels whose effect is to be compared with other treatments.

What does F stand for in ANOVA?

F = variation between sample means / variation within the samples. The best way to understand this ratio is to walk through a one-way ANOVA example. We'll analyze four samples of plastic to determine whether they have different mean strengths. You can download the sample data if you want to follow along.May 18, 2016

Is SS A positive or negative?

In contrast to our previous test statistics where positive and negative differences were possible, SS A is always positive with a value of 0 corresponding to no variation in the means. The larger the SS A, the more variation there was in the means.

Can total variation change?

In a permutation situation , the total variation (SS Total) cannot change - it is the same responses varying around the grand mean. However, the amount of variation attributed to variation among the means and in the residuals can change if we change which observations go with which group.

What does the sum of squares mean in ANOVA?

What does sum of squares mean in Anova? In the context of ANOVA, this quantity is called the total sum of squares (abbreviated SST) because it relates to the total variance of the observations. Thus: The denominator in the relationship of the sample variance is the number of degrees of freedom associated with the sample variance.

What is the sum of squares in regression?

Likewise, what is the model sum of squares? Sum of squares (SS) is a statistical tool that is used to identify the dispersion of data as well as how well the data can fit the model in regression analysis. The sum of squares got its name because they are calculated by finding the sum of the squared differences.

How to find the sum of squares?

To calculate the sum of squares, subtract each measurement from the mean, square the difference, and then add up (sum) all the resulting measurements . Likewise, what is the model sum of squares?

Why is the sum of squares called the sum of squares?

The sum of squares got its name because they are calculated by finding the sum of the squared differences. Moreover, what does mean square mean in Anova? In ANOVA, mean squares are used to determine whether factors (treatments) are significant.

What is the goal of a 2 way ANOVA?

The goal of a 2-way ANOVA is to split the to t al variation of a dependent variable (measured as Sums of Squares) into different sources of variation. This allows us to find out whether our independent variables have a significant effect on the dependent variable.

Do Type II sums of squares have an interaction effect?

Secondly, the Type II Sums of Squares do not take an interaction effect.

What is a type III sum of squares?

The Type III Sums of Squares are also called partial sums of squares again another way of computing Sums of Squares: Like Type II, the Type III Sums of Squares are not sequential, so the order of specification does not matter. Unlike Type II, the Type III Sums of Squares do specify an interaction effect.

Treatment FAQ